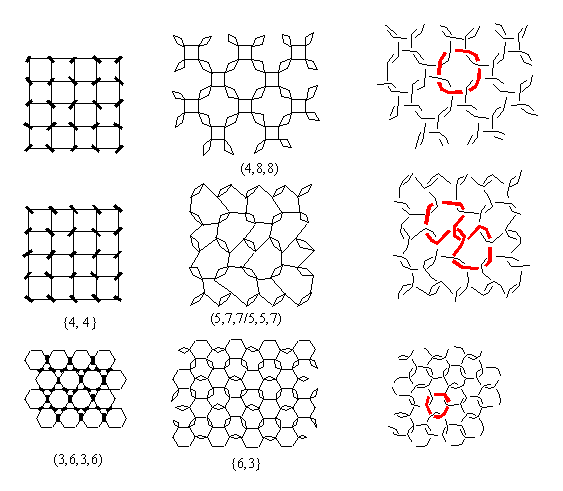
Different regular homoatomic C plane nets are discussed by T.Balaban [18]. They could be derived in the same way as the general fullerenes: by introducing digons in the vertices of 4-regular graphs or by an edge-coloring of a 3-regular graph, resulting in 4-regular one. For example, we could start from the regular tessellation {4,4}, Archimedean tiling (3,6,3,6) or 2-uniform tiling (3,4²,6;3,6,3,6) that all are 4-regular [19], and introduce digons in their vertices, or from regular tiling {6,3} that is 3-regular and color its edges (Fig. 18). From 3-regular tilings we could also derive "perfect" plane nets in the same way as before. 
  

 

For different surfaces, the necessary condition for general fullerenes follows from Euler theorem v-e+f=2-2g, where g is the genus of the surface. For the torus g=1, so accepting 5/6 restriction we conclude that for 3-regular graphs n₅= 0. In this case, the only possibility is the regular tessellation {6,3}, consisting of b²+bc+c² hexagons (where b,c are natural numbers) [5]. This tessellation we could obtain identifying opposite sides of the rectangle (Fig. 19). 
  

 

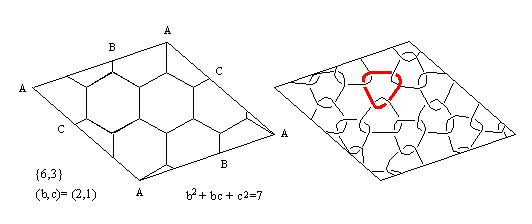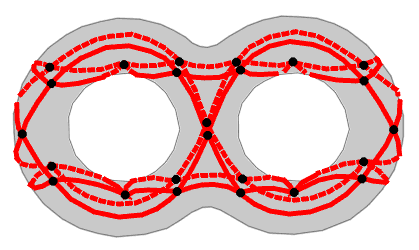
From such finite {6,3} we could simply derive the corresponding "perfect" hexagonal fullerene on torus. The proposed approach could be extended also to the double, triple, etc. torus with g=2,3,… Similar transformations of C nets from one surface to the other (e.g. from plane to cylinder, and then to torus) maybe could explain the formation of certain fullerenes and their growing process [20]. 
  

 

Accepting that the faces could be also heptagons or octagons, from the relationship 2e=3v and Euler formula, follows that n₅-n₇-2n₈= 12(1-g). For a sphere without octagons, n₅-n₇=12, and for a torus without octagons n₅=n₇ [21]. To obtain such general fullerenes with a higher degree of symmetry, we could start from different vertex-transitive structures (e.g. uniform polyhedra, stellated regular and semi-regular polyhedra or infinite polyhedra) [21]. For example, different uniform 4-valent polyhedra of the type (3,q,3,q) (q=7,8,9,10,12,18) could be used for the derivation of the corresponding "perfect" fullerenes with q-gonal holes on a double torus (g=2) (Fig. 20, q=8 [22]). For this, we use the regular vertex-bifurcation of triangular faces, transforming all of them into hexagons. In the same way, the uniform tessellations of the type (4,q,4,q) (q=5,6,8,12) or (5,10,5,10) of a double torus may result in different finite general fullerenes. The interesting classes of infinite general fullerenes with non-euclidean plane symmetry groups could be derived from the tessellations of hyperbolic plane H². For example, from the uniform tessellation (3,7,3,7) we derive the infinite perfect 6/7 fullerene in H² with heptagonal holes (Fig. 21)[21]).